4.2 The Tangent Functions
Activity 4.2.2
Show Solution
First, we will draw a picture of the situation below. We will start by solving for the length of the cables (c) Using the perspective that [latex]\sin(\theta) = \frac{opp}{hyp}[/latex] we have that
[latex]\sin(32.5) = \frac{225}{c}[/latex]
We then multiply c on both sides to get [latex]c \cdot \sin(32.5) = 225.[/latex] Dividing both sides by [latex]\sin(32.5)[/latex] yields [latex]c = \frac{225}{\sin(32.5)} \approx 418. 76[/latex].
Thus, the length of the cable should be about [latex]418.76[/latex] feet.
Now we will determine how far from the base of the tower the cables should be which is b in the figure. Using the perspective that [latex]\tan(\theta) = \frac{opp}{hyp}[/latex] we have that
[latex]\tan(\theta) = \frac{225}{b}[/latex].
We then multiply both sides of the equation by [latex]b[/latex] to get [latex]b \cdot \tan(32.5) = 225[/latex]. Dividing both sides by [latex]\tan(32.5)[/latex]yields [latex]b = \frac{225}{\tan(32.5)} \approx 353.17[/latex].
Thus, the cables should be about [latex]353.17[/latex] feet away from the base of the tower.
Examples
Surveyors are trying to determine the height of a hill relative to sea level. First they choose a point to take an initial measurement with a sextant that shows the angle of elevation from the ground to the peak of the hill is [latex]19^{\circ}[/latex]. Next they move [latex]1000[/latex] feet closer to the hill, staying at the same elevation relative to sea level, and find that the angle of elevation has increased to [latex]25^{\circ}[/latex], as pictured in Figure 4.2.11. We let [latex]h[/latex] represent the height of the hill relative to the two measurements, and [latex]x[/latex]represent the distance from the second measurement location to the “center” of the hill that lies directly under the peak
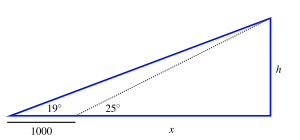
- Use the right triangle with the [latex]25^{\circ}[/latex] angle, find an equation that relates x and h.
- Using the right triangle with the [latex]19^{\circ}[/latex]angle, find a second equation that relates [latex]x[/latex] and [latex]h[/latex].
- Our work in (a) and (b) results in a system of two equations in the two unknowns [latex]x[/latex] and [latex]h[/latex]. Solve each of the two equations for [latex]h[/latex]and then substitute appropriately in order to find a single equation in the variable [latex]x[/latex].
- Solve the equation from (c) to find the exact value of [latex]x[/latex] and determine an approximately value accurate to [latex]3[/latex] decimal places.
- Use your preceding work to solve for [latex]h[/latex] exactly, plus determine an estimate accurate to [latex]3[/latex] decimal places.
- If the surveyors’ initial measurements were taken from an elevation of [latex]78[/latex] feet above seat level, how high above sea level is the peak of the hill?
Show Solution
a. Using the perspective that [latex]\tan(\theta) = \frac{opp}{hyp}[/latex], in the triangle with the [latex]25^{\circ}[/latex]angle, we have that
[latex]\tan(25^{\circ}) = \frac{h}{x}[/latex].
b. Again, we can use the fact that [latex]\tan(\theta) = \frac{opp}{hyp}[/latex] to get
[latex]\tan(19^{\circ}) = \frac{h}{x+1000}[/latex].
c. We will first solve the equation part a for h. We will start by multiplying the denominator on both sides by [latex]x[/latex].
\begin{align*}
\tan(25^{\circ}) * & = \frac{h}{x} \\
h & = x\tan(25^{\circ})
\end{align*}
Now we will solve the equation from part b by clearing the denominator.
\begin{align*}
\tan(19^{\circ}) * & = \frac{h}{x+1000} \\
h & = (x+1000)\tan(19^{\circ}) \\
\end{align*}
Now since both equations are equal to [latex]h[/latex], they must be equal to each-other. We will now set both equations equal to one another to get
[latex](x+1000)\tan(19^{\circ}) = x\tan(25^{\circ})[/latex].
d. We will solve the equation from part c for [latex]x[/latex]. We will start by distributing on the left hand side and then moving all the [latex]x[/latex] terms on one side.
\begin{align*}
(x+1000)\tan(19^{\circ}) & = x\tan(25^{\circ}) \\
x \tan(19^{\circ})+1000\tan(19^{\circ}) & = x\tan(25^{\circ}) \\
x \tan(25^{\circ}) – x\tan(19^{\circ}) & = 1000tan(19^{\circ}) \\
x (\tan(25^{\circ}) – \tan(19^{\circ})) & = 1000tan(19^{\circ}) \\
x & = \frac{1000\tan(19^{\circ})}{\tan(25^{\circ}) – \tan(19^{\circ})} \\
x & \approx 2822.819
\end{align*}
e. From part d, we have that [latex]x = \frac{1000\tan(19^{\circ})}{\tan(25^{\circ}) - \tan(19^{\circ})}[/latex]. We will plug that into one of our equations from part c when we solved for [latex]h[/latex]. We have that [latex]h = x\tan(25^{\circ}) = \frac{\tan(25^{\circ})1000\tan(19^{\circ})}{\tan(25^{\circ}) - \tan(19^{\circ})} \approx 1316.302[/latex].
f. We add the elevation to the height of the hill to get [latex]78 + 1316.302 = 1394.302[/latex] feet. The peak of the hill 1394.302 feet above sea level.
<details
